Inverse Square Law - when is a source a point source?

Any radiation training course will cover the inverse square law - as applied to protection from ionising radiation. Generally the law can be stated as follows (with respect to gamma rays or x-rays):

• If you double the distance between you and a source of ionising radiation then you will quarter the dose / dose rate at the new location.
• If you half the distance between you and a source of ionising radiation, then you will have four times the dose / dose rate at the new location.

This only applies to a point source of radiation and this is not always stated as clearly as it should be.

Do I have a point source?

Consider the following diagram.

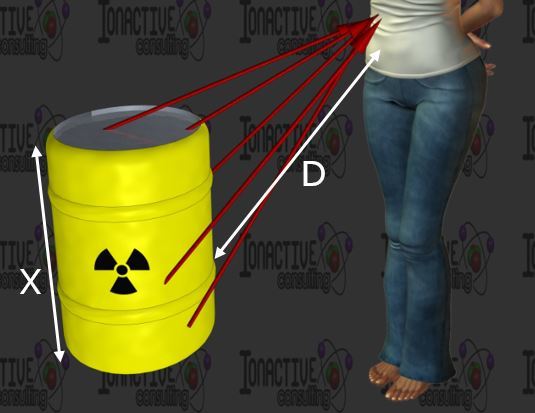
Here we have a radioactive waste drum with someone in close proximity. The rule of thumb is as follows:

The inverse square law will apply where the distance (D) from the source is at least '10*X' (where 'X' is the longest dimension of the source)

Therefore in the above diagram, regardless of the surface dose rate from the drum, its not likely it can be approximated to a point source and therefore applying the inverse square law will be unreliable. If this is a standard 200L drum then X will equal 0.85 m, and so the inverse square law might apply (at least mathematically) at distances (D) that exceed 8.5 m. However, you then need to consider the following:

• Depending on the content of drum, activity per unit volume / mass (etc), this is clearly a volume source and not a point source
• The drum contents might be 'self shielding' such that 'hot spots' measurable on the surface are not contributing in the direction of calculation (or measurement).
• Unless this is a single drum there may well be contributions from other nearby drums
• You might be too far away from the drum to detect any radiation from it

Overall all it would not be practical to treat the above case as a point source and therefore the 1/D² inverse square relationship will not apply.

Example - when is a source is a point source?

Consider a typical high activity sealed Ir-192 source as used in industrial radiography. The radioactive Ir-192 material will be in the form of one or more small pellets (generally max dimension will be 1.5 mm). These will then be loaded into a stainless steel source capsule and welded shut. Generally the longest dimension of this capsule (the 'source') will be about 5 mm (sometimes a little more). A typical activity for this example could be 740 GBq (20 Ci).

The dose rate expected at 1 m from the source will be about 84 mSv/h (line of sight with no shielding). Clearly this is a point source since 10*D (10*5 mm) = 0.05 m and this is considerably smaller than 100 cm (1 m). We can then calculate the distance at 10 cm from the source (still a point source). We can do this as follows: [(100)² / (10)²] * 84 mSv/h. This gives us 8400 mSv/h (we have rounded to keep numbers neat). This is a considerable dose rate.

We could go in nearer too. The dose rate at 5 cm, where the source may be about to lose its point source status, is about 34 Sv/hour (with rounding to keep numbers neat). That is around 0.5 Sv/minute.

Trying to calculate the dose rate on the surface of the source using the inverse square law will over estimate since its no longer a point source. However, this analysis shows that we can use inverse square law with such sources, and a clear message here is 'never pick up the source up with your fingers'! The dose rate to the fingers will be at least 0.5 Sv/minute, and on contact 10's times more - it will lead to radiation burns (deterministic effects).

If in doubt, and actually do this anyway, always monitor where you can and do not just rely on calculations. The inverse square law for true radiation point sources is a good planning tool to evaluate likely routine occupational exposure, and exposures arising from a radiation accident. Its also a useful training tool as it explains the advantage of modest distance increases, and highlights rapidly increasing dose rate as you move towards a radiation point source.